1 Answer

Carolyne · Answer 1 · 2022-01-24T22:29:06+0000

c = 22.3 km

Explanation:

You can solve this problem using cosine law:

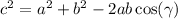
${c}^(2) = {a}^(2) + {b}^(2) - 2ab \cos( \gamma )$

where a and b are the legs of the triangle, c is the unknown length and the angle gamma is the angle opposite length c. We know that

a = 50 km

b= 52.4 km

gamma = 25°

c^2 = (50 km)^2 + (52.4 km)^2 -2(50)(52.4)cos25°

= 496.707 km^2

or

c = 22.3 km

Please log in or register to add a comment.