Question

1. Pam has a mass of 48.3 kg and she is at rest on

smooth, level, frictionless ice. Pam straps on
a rocket pack. The rocket supplies a constant
force for 27.3 m and Pam acquires a speed of
62 m/s.
What is the magnitude of the force?
Answer in units of N.

2. What is Pam’s final kinetic energy?
Answer in units of J.

3. A child and sled with a combined mass of 55.7
kg slide down a frictionless hill that is 11.3 m
high at an angle of 29 ◦
from horizontal.
The acceleration of gravity is 9.81 m/s

3. If the sled starts from rest, what is its speed
at the bottom of the hill?
Answer in units of m/s

Answer 1

1. F = 3400 N = 3.4 KN

2.
`K.E_f=92832.6\ J = 92.83\ KJ`

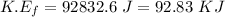
3. v = 14.9 m/s

Step-by-step explanation:

1.

First, we will calculate the acceleration of Pam by using the third equation of motion:

`2as = v_f^2-v_i^2`

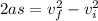
where,

a = acceleration = ?

s = distance = 27.3 m

vf = final speed = 62 m/s

vi = initial speed = 0 m/s

Therefore,

`2a(27.3\ m) = (62\ m/s)^2-(0\ m/s)^2\\\\a = 70.4\ m/s^2`

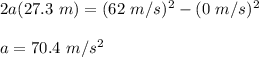
Now, we will calculate the force by using Newton's Second Law of Motion:

F = ma

F = (48.3 kg)(70.4 m/s²)

F = 3400 N = 3.4 KN

2.

Final kinetic energy is given as:

`K.E_f = (1)/(2)mv_f^2\\\\K.E_f = (1)/(2) (48.3\ kg)(62\ m/s)^2`

`K.E_f=92832.6\ J = 92.83\ KJ`

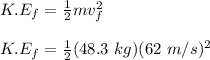
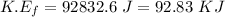
3.

According to the law of conservation of energy:

`Potential\ Energy\ at\ top = Kinetic\ Energy\ at\ bottom\\mgh = (1)/(2)mv_2 \\\\v = √(2gh)`

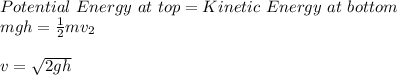
where,

v = speed at bottom = ?

g = acceleration due to gravity = 9.81 m/s²

h = height at top = 11.3 m

Therefore,

`v = √((2)(9.81\ m/s^2)(11.3\ m))`

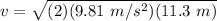
v = 14.9 m/s