Question

A person carries a plank of wood 1.9 m long with one hand pushing down on it at one end with a force F1 and the other hand holding it up at 49 cm from the end of the plank with force F2. If the plank has a mass of 14 kg and its center of gravity is at the middle of the plank, what are the magnitudes of the forces F1 and F2? (The distance of 49 cm is measured from the location of F1.)

Answer 1

Given :

mass of the plank, m = 14 kg

length of the plank, l = 1.9 m

Distance between
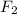`$F_2$` and the end at which
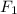`$F_1$` is acting,
`$r_2$` = 0.49 m

Torque exerted by the weight of the plank is given by :

`$\tau_w= r_(\perp) * F$`

`$=-(1.9)/(2) * 14 * 9.8$`

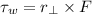
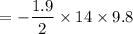
= -130 Nm

The torque exerted by
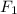`$F_1$` is

`$\tau_1= r_(1) * F_1$`

`$= 0 * F_1$`

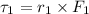
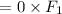
= 0

Torque exerted by
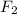`$F_2$` is

`$\tau_2= r_(2) * F_2$`

`$= 0.49 * F_2$`

`$= 0.49 F_2$`

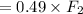
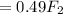
Since the system is in equilibrium, the zero rotational acceleration occurs when the net external torque on the system is zero, i.e.

`$\sum \tau = 0$`

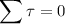
Therefore, we get

`$$\sum \tau= \tau_1+\tau_2+\tau_w`

`$0= 0+0.49F_2+(-130)$`

`$F_2=(130)/(0.49)$`

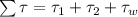
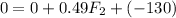
= 265 N (approx)

We know that zero linear acceleration occurs when the net external force is zero on the system to achieve equilibrium, i.e.

`$\sum F = 0$`

`$\sum F = -F_1+F_2-mg=0$`

`$F_1=F_2-mg$`

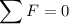
= 265 - (14 x 9.8)

= 128 N (approx)