Answer:
They should sample the results of 1990 patient results.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the z-score that has a p-value of
 .
.
The margin of error is of:

A reproductive clinic had a success rate of 25% of patients with live births in 2008.
This means that
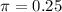
99% confidence level
So
 , z is the value of Z that has a p-value of
, z is the value of Z that has a p-value of
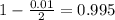 , so
, so
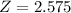 .
.
If they want their estimate to be within 2.5%, how many patient's results should they sample if they plan to use a 99% confidence interval?
They should sample n patients.
n is found for
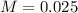 . So
. So


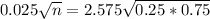

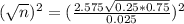
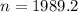
Rounding up:
They should sample the results of 1990 patient results.