Question

the auto parts department of an automotive dealership sends out a mean of 66 special orders daily. what is the probability that, for any day, the number of special orders sent out will be no more than 33

Answer 1

0% probability that, for any day, the number of special orders sent out will be no more than 33

Explanation:

Normal Probability Distribution:

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Mean of 66 special orders daily

This means that
`\mu = 66`. We have the mean during a time interval, which means that the number of special orders follow a Poisson distribution, which has
`\sigma = √(\mu) = √(66)`

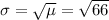
What is the probability that, for any day, the number of special orders sent out will be no more than 33?

Since the Poisson distribution, which is discrete, is approximated to the normal(which is continuous), we have to use continuity correction, and thus, this probability is P(X < 33 - 0.5) = P(X < 32.5), which is the p-value of Z when X = 32.5.

`Z = (X - \mu)/(\sigma)`

`Z = (32.5 - 66)/(√(66))`

`Z = -4.12`

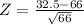
`Z = -4.12` has a p-value of 0.

0% probability that, for any day, the number of special orders sent out will be no more than 33