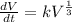Answer:
The differential equation becomes -
![(dV)/(dt) = k\sqrt[3]{V}](https://img.qammunity.org/2022/formulas/mathematics/college/s4ebjpny9kywoj5781g54jyosghtshh8mn.png) i.e.
i.e.
Explanation:
Given - The rate of change of the volume V of water in a tank with respect to time t is directly proportional to the cubed root of the volume.
To find - Write a differential equation that describes the relationship.
Proof -
Rate of change of volume V with respect to time t is represented by
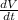
Now,
Given that,
The rate of change of the volume V of water in a tank with respect to time t is directly proportional to the cubed root of the volume.
⇒
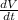 ∝
∝
![\sqrt[3]{V}](https://img.qammunity.org/2022/formulas/mathematics/college/3xer2nk91r90mhz3kxo34yiszwt5lnhg40.png)
Now,
We know that, when we have to remove the Proportionality sign , we just put a constant sign.
Let k be any constant.
So,
The differential equation becomes -
![(dV)/(dt) = k\sqrt[3]{V}](https://img.qammunity.org/2022/formulas/mathematics/college/s4ebjpny9kywoj5781g54jyosghtshh8mn.png) i.e.
i.e.