Question

g A 12,000 m3/day treatment plant has a rectangular sedimentation basin with dimensions 12 meters wide, 3 meters deep, and 25 meters long. Will particles with a settling velocity of 6 x 10-3 m/s be removed in this basin

Answer 1

The settling velocity of the particles (Vs) is greater than the overflow rate (V₀), thus the particles will settle out and will be removed.

Step-by-step explanation:

Given;

volumetric flow rate of the treatment, Q₀ = 12,000 m³/day

length of the rectangular tank, L = 25 m

width of the tank, W = 12 m

height of the tank, H = 3 m

settling velocity of the particles,
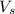`V_s` = 6 x 10⁻³ m/s

The overflow rate of the sediments are calculated as follows;

`V_o = (Q_o)/(A_s)`

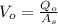
where;

As is the surface area of the tank, m²

Q₀ is the flow rate, m³/s

As = 2LW + 2LH + 2WH

As = (2 x 25 x 12) + (2 x 25 x 3) + (2 x 12 x 3)

As = 822 m²

`Q_0 (m^3/s)= (12,000 \ m^3)/(day) * (1 \ day)/(24 \ hr) * (1 \ hour)/(60 \ \min) * (1 \ \min)/(60 \ s) = (12,000)/(24 * 60 * 60) (m^3/s)= (12,000)/(86,400) \ m^3/s\\\\Q_o = 0.139 \ m^3/s`

The overflow rate;

`V_o = (Q_0)/(A_s) = (0.139)/(822) = 1.69 * 10^(-4) \ m/s`

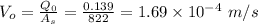
The settling velocity of the particles (Vs) is greater than the overflow rate (V₀), thus the particles will settle out and will be removed.