Question

A certain regression line equation has the slope equal to 326.07 and an intercept that is equal to 8.19. What will be the predicted score for the dependent variable if the independent variable is 608.6?

Answer 1

The predicted score for the dependent variable will be of 198,454.4.

Explanation:

Equation of a regression line:

The equation of a regression line has the following format:

`y = ax + b`

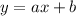
In which a is the slope and b is the intercept. x is the independent variable while y is the dependent variable.

A certain regression line equation has the slope equal to 326.07 and an intercept that is equal to 8.19.

So
`y = 326.07x + 8.19`

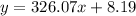
What will be the predicted score for the dependent variable if the independent variable is 608.6?

y when x = 608.6. So

`y = 326.07*608.6 + 8.19 = 198454.4`

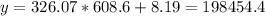
The predicted score for the dependent variable will be of 198,454.4.