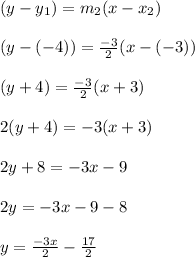Question

Write the slope-intercept form of the equation of the line that passes thrqugh (-4, -3) and is

perpendicular to the graph of 2x - 3y = -5.

Answer 1

Explanation:

Equation of the line y = mx + c

The new line is perpendicular to 2x - 3y = -5

- 3y = - 5 - 2x

3y = 2x + 5

y =
`(2x)/(3) + (5)/(3)`

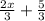
Since the lines are perpendicular to each other :
`m_(1) . m_(2) = -1`

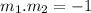
where
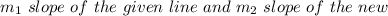`m_(1) \ slope \ of \ the \ given \ line \ and \ m_(2) \ slope \ of \ the \ new \line\\`.

Slope of the given line

`m_(1) = (2)/(3)`

Slope of the new line

`\\(2)/(3).m_(2) = -1\\ m_(2) = -1 . (3)/(2) = (-3)/(2)`

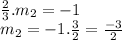
The equation to the new line passing through (-4, -3) and perpendicular to 2x - 3y = -5

`(y - y_(1)) = m_(2)(x-x_(2) )\\\\(y - (-4)) = (-3)/(2)(x - (-3)) \\\\(y + 4) = (-3)/(2) (x+ 3)\\\\2(y +4) = -3(x+3)\\\\2y + 8 = -3x -9 \\\\2y = -3x - 9 -8\\\\y = (-3x)/(2) - (17)/(2)`