Answer:
The building is 746.666 meters high.
Step-by-step explanation:
We know the total velocity of the rock (
 ), in meters per second, and its horizontal speed (
), in meters per second, and its horizontal speed (
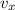 ), in meters per second, at the moment right before its impact. It should be noted that the rock is experimenting a parabolic motion, which is the combination of a horizontal motion at constant speed and a free fall, which is an uniform accelerated motion due to gravity.
), in meters per second, at the moment right before its impact. It should be noted that the rock is experimenting a parabolic motion, which is the combination of a horizontal motion at constant speed and a free fall, which is an uniform accelerated motion due to gravity.
The final vertical speed of the rock (
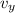 ), in meters per second, is determined by the following Pythagoric formula:
), in meters per second, is determined by the following Pythagoric formula:
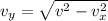 (1)
(1)
If we know that
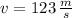 and
and
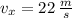 , then the final vertical speed is:
, then the final vertical speed is:
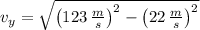
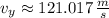
Now we determine the height of the building (
 ), in meters, by the use of the following kinematic expression:
), in meters, by the use of the following kinematic expression:
 (2)
(2)
Where:
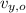 - Initial vertical speed of the rock, in meters per second.
- Initial vertical speed of the rock, in meters per second.
 - Gravitational acceleration, in meters per square second.
- Gravitational acceleration, in meters per square second.
If we know that
 ,
,
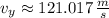 and
and
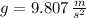 , then the height of the building is:
, then the height of the building is:

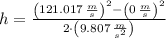
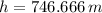
The building is 746.666 meters high.