Question

A certain FM radio station broadcasts electromagnetic waves at a frequency of 60,500,000 Hz. These waves travel at a velocity of 300,000,000 m/s. What is the wavelength of these radio waves?

A. 4.96 m

B.0.2 m

C. 360,500,000 m

Answer 1

The wavelength
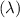`(\(\lambda\))` of a radio wave with a frequency of 60,500,000 Hz and velocity of 300,000,000 m/s is approximately 4.96 meters. So the correct option is A.

The wavelength
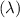`(\(\lambda\))` of a wave can be calculated using the formula:

`\[ \lambda = (v)/(f) \]`

where:

-
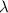`\(\lambda\)` is the wavelength,

-
`\(v\)` is the velocity of the wave,

-
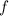`\(f\)` is the frequency of the wave.

In this case, the frequency
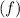`(\(f\))` is given as 60,500,000 Hz, and the velocity
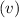`(\(v\))` is given as 300,000,000 m/s. Plugging in these values:

`\[ \lambda = \frac{300,000,000 \, \text{m/s}}{60,500,000 \, \text{Hz}} \]`

Calculating this gives:

`\[ \lambda \approx 4.9587 \, \text{m} \]`

So, the wavelength is approximately 4.96 meters.

Therefore, the correct answer is :A. 4.96 m

Answer 2

Wavelength of radio wave = 4.96 meter (Approx.)

Step-by-step explanation:

Given:

Frequency of radio wave = 60,500,000 Hz

Velocity of radio waves = 300,000,000 m/s

Find:

Wavelength of radio wave

Computation:

Wavelength = Velocity / frequency

Wavelength of radio wave = Velocity of radio waves / Frequency of radio wave

Wavelength of radio wave = 300,000,000 / 60,500,000

Wavelength of radio wave = 4.9586

Wavelength of radio wave = 4.96 meter (Approx.)