Answer:
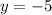
Explanation:
Given
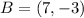
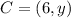
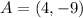
Required
The y coordinate of C
Since A, B and C are on the same line, the slope of AB and the slope of AC will be the same.
Slope (m) is calculated as:

For AC
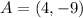
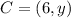
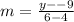

For AB
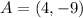
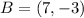
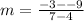


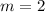
So, we have:
 and
and
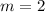

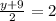
Multiply both sides by 2
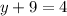
Solve for y

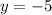
Hence, the y coordinate of C is -5