Question

A.

Suppose that 61% of residents in a certain city own at least one pet. You select a random sample of 40 city
residents. Let Ø - the proportion of city residents in your sample that own at least one pet. What is the
standard deviation of the sampling distribution of P and what does it mean?
In SRSs of size 40 from the population of city residents, the proportion of residents that
own at least one pet in the sample will typically vary by about 0.006 from the true
proportion of 0.61.
b. In SRSs of size 40 from the population of city residents, the proportion of residents that
own at least one pet in the sample will typically vary by about 0.077 from the true
proportion of 0.61.
c. In SRSs of size 40 from the population of city residents, the proportion of residents that
own at least one pet in the sample will typically vary by about 0.238 from the true
proportion of 0.61.
d. In SRS of size 40 from the population of city residents, the proportion of residents that
own at least one pet in the sample will typically vary by about 0.488 from the true
proportion of 0.61.

Answer 1

b. In SRSs of size 40 from the population of city residents, the proportion of residents that own at least one pet in the sample will typically vary by about 0.077 from the true proportion of 0.61.

Explanation:

Central Limit Theorem

The Central Limit Theorem establishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

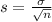For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean
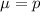`\mu = p` and standard deviation
`s = \sqrt{(p(1-p))/(n)}`

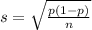
Suppose that 61% of residents in a certain city own at least one pet.

This means that
`p = 0.61`

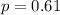
You select a random sample of 40 city residents.

This means that
`n = 40`

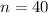
What is the standard deviation of the sampling distribution of P and what does it mean?

`s = \sqrt{(p(1-p))/(n)} = \sqrt{(0.61*0.39)/(40)} = 0.077`

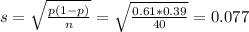
This means that in samples of size 40 the proportion of residents that own at least one pet in the sample will typically vary by about 0.077 from the true proportion of 0.61, which means that the correct answer is given by option b.