Question

Which statement correctly compares the two functions on the interval [-1,2]?

Answer 1

Option A.

Explanation:

Function f:

For x between -1 and 2, the values of f(x) increase, which means that f(x) is increasing.

Function g:

`g(x) = -18((1)/(3))^x + 2`

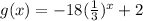
Between -1 and 2:

`g(-1) = -18((1)/(3))^(-1) + 2 = -18*3 + 2 = -54 + 2 = -52`

`g(0) = -18((1)/(3))^(0) + 2 = -18*1 + 2 = -18 + 2 = -16`

`g(1) = -18((1)/(3))^(1) + 2 = -18*(1)/(3) + 2 = -6 + 2 = -4`

`g(2) = -18((1)/(3))^(2) + 2 = -18*(1)/(9) + 2 = -2 + 2 = 0`

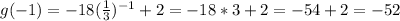
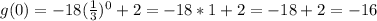
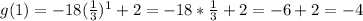
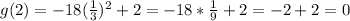
Both are increasing.

However, g starts with a lower value, and finishes with a higher value, which means that function g increases at a faster average rate, and the correct answer is given by option A.