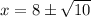Question

Solve the equation x^2 - 16x+ 54 = 0 by completing the square.

Fill in the values of a and b to complete the solutions.

Answer 1

Explanation:

Answer 2

`x = 8\±\sqrt{10`

`a =8`
`b = 10`

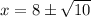
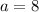
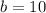
Explanation:

Given

`x^2 - 16x + 54 = 0`

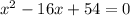
Required

Complete the square

`x^2 - 16x + 54 = 0`

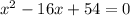
Subtract 54 from both sides

`x^2 - 16x + 54 -54= 0-54`

`x^2 - 16x = -54`

--------------------------------------------------

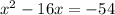
Take half of - 16

`(-16/2) = -8`

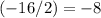
Square the result

`(-8)^2 = 64`

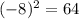
Add the squared result to both sides of the equation

--------------------------------------------------

So, we have:

`x^2 - 16x +64= -54+64`

`x^2 - 16x +64= 10`

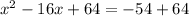
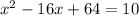
Expand

`x^2 - 8x - 8x + 64 = 10`

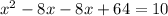
Factorize

`x(x - 8) - 8(x - 8) = 10`

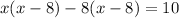
Factor out x - 8

`(x - 8)(x - 8) = 10`

`(x - 8)^2 = 10`

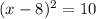
Take square roots

`x - 8 = \±\sqrt{10`

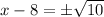
Solve for x

`x = 8\±\sqrt{10`

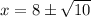
We have:

`x = a - √(b)`

`x = a + √(b)`

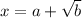
By comparing the above with:
`x = 8\±\sqrt{10`