Question

What is the new pressure of 150 mL of a gas that is compressed to 50 mL when the original pressure was 3.0 ATM and the temperature is held constant

Answer 1

`\boxed {\boxed {\sf P_2= 9 \ atm}}`

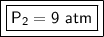
Step-by-step explanation:

Since the temperature is held constant, we only need to focus on the volume and pressure. We will use Boyle's Law, which states the volume of a gas is inversely proportional to the pressure. The formula is:

`P_1V_1=P_2V_2`

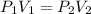
Originally, the gas had a volume of 150 milliliters and a pressure of 3.0 atmospheres. We can substitute these values into the left side of the equation.

`3.0 \ atm * 150 \ mL = P_2V_2`

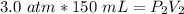
The original gas was compressed to a volume of 50 milliliters, but we don't know the volume.

`3.0 \ atm *150 \ mL= P_2 * 50 \ mL`

Now, we need to solve for the new pressure (P₂). Multiply on the left side first.

`450 \ atm*mL= P_2 * 50 \ mL`

Since we are solving for the pressure, we need to isolate the variable. It is being multiplied by 50 mL. The inverse of multiplication is division. Divide both sides by 50 mL.

`(450 \ atm*mL)/(50 \ mL)= (P_2 * 50 \ mL)/(50 \ mL)`

`(450 \ atm*mL)/(50 \ mL)= P_2`

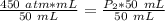
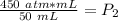
The units of milliliters will cancel.

`(450 \ atm)/(50 )= P_2`

`9 \ atm =P_2`

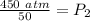
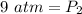
The new pressure is 9 atmospheres.