Question

Which polynomial function has a leading coefficient of 2, root-5 with multiplicity 3, and root 12 with multiplicity 1?

Of(x) = 2(x - 5)(x - 5)(x - 5)(x + 12)
Of(x) = 2(x + 5)(x + 5)(x + 5)(x - 12)
O f(x) = 3(x - 5)(x - 5)(x + 12)
Of(x) = 3(x + 5)(x + 5)(x - 12)

Answer 1

The polynomial function that has a leading coefficient of 2, a root of 5 with a multiplicity of 3, and a root of 12 with a multiplicity of 1 is choice 2.

Explanation:

A leading coefficient of 2 means that the number multiplying all the binomial would be 2, so you know it is not choices 3 and 4. A root of -5 with a multiplicity of 3 means that when you replace x with -5, three binomials will result in 0, which helps eliminate the first choice. A root of 12 with a multiplicity of 1 means that when you replace x with 12, you will have a binomial that results in 0. Using all of this information, you'll get that the polynomial that has a leading coefficient of 2, a root of -5 with a multiplicity of 3, and a root of 12 with a multiplicity of 1 is
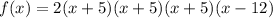`f(x)=2(x+5)(x+5)(x+5)(x-12)`.

Answer 2

Of(x) = 2(x + 5)(x + 5)(x + 5)(x - 12) or Choice 2

Explanation:

The last two answers get eliminated because their leading coefficients aren't 2. The first option is wrong because if you plug in -5 and +12 into x the function won't equal 0. So the correct answer is the Second answer: Of(x) = 2(x + 5)(x + 5)(x + 5)(x - 12)