Question

A tunnel is built in form of a parabola. The width at the base of tunnel is 7 m. On

the ceiling 3 m from each end of the base there are light fixtures. The height to light
fixtures is 4 m. A trailer truck carrying cars is 4 m wide and 2.8 m high is going to drive
through the tunnel? Is this possible? (Give full algebraic solution.

Answer 1

Given:

The width at the base of parabolic tunnel is 7 m.

The ceiling 3 m from each end of the base there are light fixtures.

The height to light fixtures is 4 m.

To find:

Whether it is possible a trailer truck carrying cars is 4 m wide and 2.8 m high is going to drive through the tunnel.

Solution:

The width at the base of tunnel is 7 m.

Let the graph of the parabola intersect the x-axis at x=0 and x=7. It means x and (x-7) are the factors of the height function.

The function of height is:

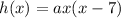`h(x)=ax(x-7)` ...(i)

Where, a is a constant.

The ceiling 3 m from each end of the base there are light fixtures and the height to light fixtures is 4 m. It means the graph of height function passes through the point (3,4).

Putting x=3 and h(x)=4 in (i), we get

`4=a(3)((3)-7)`

`4=a(3)(-4)`

`(4)/((3)(-4))=a`

`-(1)/(3)=a`

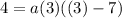
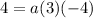
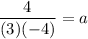
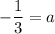
Putting
`a=-(1)/(3)`, we get

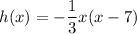`h(x)=-(1)/(3)x(x-7)` ...(ii)

The center of the parabola is the midpoint of 0 and 7, i.e., 3.

The width of the truck is 4 m. If is passes through the center then the truck must m 2 m on the left side of the center and 2 m on the right side of the center.

2 m on the left side of the center is x=1.5.

A trailer truck carrying cars is 4 m wide and 2.8 m high is going to drive through the tunnel is possible if h(1.5) is greater than 2.8.

Putting x=1.5 in (ii), we get

`h(1.5)=-(1)/(3)(1.5)(1.5-7)`

`h(1.5)=-(0.5)(-5.5)`

`h(1.5)=2.75`

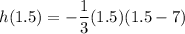
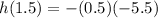
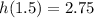
It is clear that h(1.5)<2.8, therefore the trailer truck carrying cars is 4 m wide and 2.8 m high is going to drive through the tunnel is not possible.