Question

Find the probability of at least one

failure in five trials of a binomial
experiment in which the probability of
success is 30%.
Round to the nearest tenth of a
percent.

Answer 1

99.8% probability of at least one failure.

Explanation:

Binomial probability distribution

The binomial probability is the probability of exactly x successes on n repeated trials, and X can only have two outcomes.

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

In which
`C_(n,x)` is the number of different combinations of x objects from a set of n elements, given by the following formula.

`C_(n,x) = (n!)/(x!(n-x)!)`

And p is the probability of X happening.

Probability of success is 30%.

This means that
`p = 0.3`

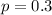
Five trials:

This means that
`n = 5`

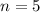
Find the probability of at least one failure in five trials of a binomial experiment in which the probability of success is 30%.

Less than five sucesses, which is:

`P(X < 5) = 1 - P(X = 5)`

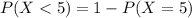
In which

`P(X = x) = C_(n,x).p^(x).(1-p)^(n-x)`

`P(X = 5) = C_(5,5).(0.3)^(5).(0.7)^(0) = 0.002`

`P(X < 5) = 1 - P(X = 5) = 1 - 0.002 = 0.998`

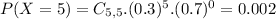
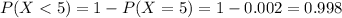
0.998*100% = 99.8%

99.8% probability of at least one failure.