Answer:

Step-by-step explanation:
Since we are dealing with temperature and pressure, we must use Boyle's Law. This states that the pressure is inversely proportional to the volume. The formula is:
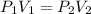
Where P is the pressure and V is the volume.
We know the original pressure (P₁) of the gas is 1.20 atmospheres and the volume (V₁) is 2.45 liters.

We also know the gas is contracted to a new volume of 2.20 liters (V₂) , but we do not know the pressure (P₂). We can use x.
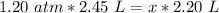
We can solve the left side of the equation and multiply.
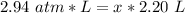
We are trying to find the new pressure (x), so we must isolate the variable. It is being multiplied by 2.20 liters. The inverse of multiplication is division, so we divide both sides by 2.20 L.
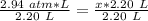
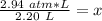
The units of liters cancel.

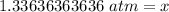
The original measurements have 3 significant figures, so our answer should have the same. For the number we found, that is the hundredth place.
The 6 in the thousandth place tells us to leave the 3 in the hundredth place.
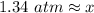
The new pressure is approximately 1.34 atmospheres.