Answer:
p = 22.5 cm
Step-by-step explanation:
For this exercise we must use the equation of the constructor
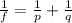
where f is the focal length, p and q are the distance to the object and the image respectively.
Let's start with the far point, the object is very far away (p = ∞) and the image must be formed at the far point of view of the person q = 45.0 cm
since the image is on the same side as the object according to the sign convention the distance is negative
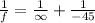
f = -45.0 cm
now let's use the near point (q = 15.0 cm) at what distance the object should be
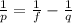
 1 / p = 1 / -45 - 1 / -15
1 / p = 1 / -45 - 1 / -15
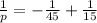 1 / p = -1/45 + 1/15
1 / p = -1/45 + 1/15
 = 0.0444
= 0.0444
p = 22.5 cm
this is the closest distance you can see an object clearly