Question

Consider a game in which players draw playing cards one at a time from a standard 52-card deck. If a player draws a face card (a jack, a queen, or a king), the player is awarded 16 points. Any other card drawn earns the player 3 points. What is the expected value of drawing a card in this game on the first turn?

A. 3.7 points B. 6 points C. 6.3 points D. 9.5 points

Answer 1

Expected value of drawing a card in this game on the first turn = 6

Explanation:

Given - Consider a game in which players draw playing cards one at a time from a standard 52-card deck. If a player draws a face card (a jack, a queen, or a king), the player is awarded 16 points. Any other card drawn earns the player 3 points.

To find - What is the expected value of drawing a card in this game on the first turn?

Formula used -

Expected value, E[x] = ∑ x p(x)

where p(x) is the probability

Proof -

Total cards in a standard deck = 52

Total face cards = 12 (a jack, a queen, or a king)

Other cards = 40

Now,

Probability of getting a face card =
`(12)/(52)`

Probability of getting a other card =
`(40)/(52)`

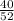
So,

Expected value, E[x] = ∑ x p(x)

= (16)(
`(12)/(52)`) + (3)(
`(40)/(52)`)

=
`(192)/(52) + (120)/(52)`

=
`(312)/(52)`

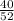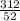
= 6

∴ we get

Expected value of drawing a card in this game on the first turn = 6

So,

The correct option is - B. 6 points