Answer:
0.9 = 90% probability that it arrived during the last 30 seconds of the 5-minute period.
Explanation:
The car is equally as likely to arrive during each second of the interval, which means that the uniform distribution is used to solve this question.
A distribution is called uniform if each outcome has the same probability of happening.
The uniform distribution has two bounds, a and b, and the probability of finding a value higher than x is given by:
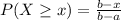
5-minute period
This means that
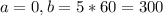
Find the probability that it arrived during the last 30 seconds of the 5-minute period.
300 - 30 = 270. So
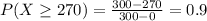
0.9 = 90% probability that it arrived during the last 30 seconds of the 5-minute period.