Question

A golf club rotates 215 degrees and has a length (radius) equal to 29 inches. The time it took to swing the club was 0.8 seconds. What is the average linear velocity (inches/second) of the golf club

Answer 1

The average linear velocity (inches/second) of the golf club is 136.01 inches/second

Step-by-step explanation:

Given;

length of the club, L = 29 inches

rotation angle, θ = 215⁰

time of motion, t = 0.8 s

The angular speed of the club is calculated as follows;

`\omega = ((\theta)/(360) * 2\pi, \ rad) * (1)/(t) \\\\\omega = ((215)/(360) * 2\pi, \ rad) * (1)/(0.8 \ s) \\\\\omega = 4.69 \ rad/s`

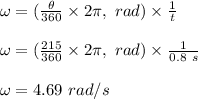
The average linear velocity (inches/second) of the golf club is calculated as;

v = ωr

v = 4.69 rad/s x 29 inches

v = 136.01 inches/second

Therefore, the average linear velocity (inches/second) of the golf club is 136.01 inches/second