Question

The Office of Student Services at a large western state university maintains information on the study habits of its full-time students. Their studies indicate that the mean amount of time undergraduate students study per week is 20 hours. The hours studied follows the normal distribution with a population standard deviation of six hours. Suppose we select a random sample of 150 current students. What is the probability that the mean of this sample is between 19.25 hours and 21.0 hours

Answer 1

0.8254 = 82.54% probability that the mean of this sample is between 19.25 hours and 21.0 hours

Explanation:

To solve this question, we need to understand the normal probability distribution and the central limit theorem.

Normal Probability Distribution:

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Central Limit Theorem

The Central Limit Theorem estabilishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

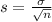For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

Mean of 20 hours, standard deviation of 6:

This means that
`\mu = 20, \sigma = 6`

Sample of 150:

This means that
`n = 150, s = (6)/(√(150))`

What is the probability that the mean of this sample is between 19.25 hours and 21.0 hours?

This is the p-value of Z when X = 21 subtracted by the p-value of Z when X = 19.5. So

X = 21

`Z = (X - \mu)/(\sigma)`

By the Central Limit Theorem

`Z = (X - \mu)/(s)`

`Z = (21 - 20)/((6)/(√(150)))`

`Z = 2.04`

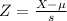
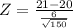
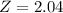
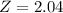`Z = 2.04` has a p-value of 0.9793

X = 19.5

`Z = (X - \mu)/(s)`

`Z = (19.5 - 20)/((6)/(√(150)))`

`Z = -1.02`

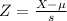
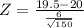
`Z = -1.02` has a p-value of 0.1539

0.9793 - 0.1539 = 0.8254

0.8254 = 82.54% probability that the mean of this sample is between 19.25 hours and 21.0 hours