Solve for a and b in the following circles: A)

Question

asked Nov 22, 2022 19.2k views

1 Answer

Ynkr · Answer 1 · 2022-11-27T04:48:08+0000

Given:

A quadrilateral inscribed in a circle.

To find:

The values of a and b.

Solution:

A quadrilateral inscribed in a circle. So, the quadrilateral is a cyclic quadrilateral.

We know that the sum of the opposite angles of a cyclic quadrilateral is 180 degrees.

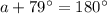
$a+79^\circ=180^\circ$

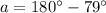
$a=180^\circ-79^\circ$

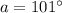
$a=101^\circ$

We know that the measure of arc is twice of measure of subtended angle on that arc.

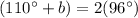
$(110^\circ+b)=2(96^\circ)$

$110^\circ+b=192^\circ$

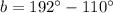
$b=192^\circ-110^\circ$

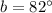
$b=82^\circ$

Therefore, the value of a is 101 degrees and the value of b is 82 degrees.