Question

A teacher is making a history test composed of the same number of multiple-choice questions as short-answer questions. She estimates it will take students an average of 2 minutes to complete each multiple-choice question and an average of 3.5 minutes to complete each short-answer question. Write an inequality to determine how many questions, n, the teacher can include if the test must take students less than 45 minutes to complete.

Answer 1

The inequality is
`5.5n \leq 45`

Explanation:

Total time:

The total time to complete the test is the sum of the number of multiple-choice questions multiplied by the time it takes to solve each and the number of short-answer questions multiply by the time it takes to solve each.

In this question:

Same number(n) of both.

Multiple-choice takes 2 minutes, short-answer takes 3.5 minutes. The total time is given by:

`T = 2n + 3.5n = 5.5n`

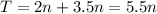
Write an inequality to determine how many questions, n, the teacher can include if the test must take students less than 45 minutes to complete.?

This means that:

`T \leq 45`

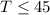
So

`5.5n \leq 45`

The inequality is
`5.5n \leq 45`