Question

In 2004, a survey on computer crime indicated that 7% of reported unauthorized uses of business Web sites came from inside the company. In a survey taken two years later, it was found that 7 of a sample of 163 reported unauthorized uses of business Web sites came from inside the company. a) Conduct an appropriate hypothesis test to check if the proportion of reported unauthorized uses of business Web sites that came from inside the company has changed since 2004. Use the significance level of 0.05. State your conclusion. (5 Points) b) Find the p-value of the test and confirm that the conclusion based on the p-value agrees with the conclusion in part (a). (2 Points)

Answer 1

a) H0 : P = 0.07

Ha : P ≠ 0.07

b) p -value = 0.1770

Explanation:

P = 7% = 0.07

x (result ) = 7 , n = 163

p = x / n = 7 / 163 = 0.043

a) Using a significance level ( ∝ ) of 0.05, estimate the appropriate hypothesis

H0 : P = 0.07

Ha : P ≠ 0.07

conduct a Z- test statistic

Z = ( p - P ) /
`\sqrt{(P(1-P))/(n) }`

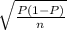
= ( 0.043 - 0.07 ) /
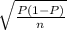`\sqrt{(P(1-P))/(n) }` = - 1.35

Critical value ( Z₀.₀₂₅ ) = ± 1.96

we fail to reject H0 given that | z | < Zcritical because there is not enough evidence to conclude that proportion change

b) Determine the p-value of the test

P-value = P ( | Z | > Z )

= 2 * P ( Z < -1.35 )

= 0.1770

The p-value ( 0.1770 ) > ∝ ( 0.05 ) hence we fail to reject H0 ( i.e. the conclusion agrees with part a above )