Question

For a typical human aorta, the diameter of the lumen is 30 mm and the thickness of the wall is 4 mm. Assuming a blood density of 1.06 gm/cc and a viscosity of 0.035 Poise, calculate the Womersley number if the heart rate is 70 bpm. Diameters for the carotid and femoral arteries of a typical human are about 0.8 cm and 0.5 cm, respectively. Compute the Womersley numbers for each of the arterial segments for the same heart rate and explain if the flows can be assumed steady or not.

Answer 1

Womersley numbers : 22.25 , 5.96 , 3.72

Step-by-step explanation:

Calculate the Womersley number if the heart rate = 70 bpm

For the Human Aorta

∝ = r
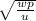`\sqrt{(wp)/(u) }` ------ ( 1 )

r = radius = 15 mm = 0.015 m

w = 2πf = 2 * π * 70 = 439.82

p = density of blood = 1060 kg/m^3

u = 0.035 P = 3.5 cp = 3.5 * 10^-3 Ns/m^2

Back to equation ( 1 )

∝ = 0.015 *
`\sqrt{(439.82*1060)/(60*3.5*10^(-3) ) }` = ( 0.015 * √466209.2 / 0.21 ) = 22.35

For Carotid artery

r = 0.8 cm / 2 = 0.4 cm = 0.004 m

w = 2πf = 439.82

Womersley number = ( 0.004 * √466209.2 / 0.21 )

= 0.004 * 1489.981 = 5.96

For Femoral artery

r = 0.5 cm / 2 = 0.25 cm = 0.0025 m

Womersley number = 0.0025 * √466209.2 / 0.21 )

= 0.0025 * 1489.981 = 3.72

The flow is unsteady because of the varying Womersley numbers