Answer:
The equation of the parabola is
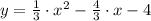 , whose real vertex is
, whose real vertex is
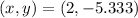 , not
, not
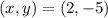 .
.
Explanation:
A parabola is a second order polynomial. By Fundamental Theorem of Algebra we know that a second order polynomial can be formed when three distinct points are known. From statement we have the following information:
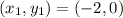 ,
,
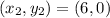 ,
,
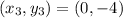
From definition of second order polynomial and the three points described above, we have the following system of linear equations:
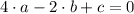 (1)
(1)
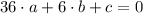 (2)
(2)
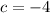 (3)
(3)
The solution of this system is:
 ,
,
 ,
,
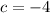 . Hence, the equation of the parabola is
. Hence, the equation of the parabola is
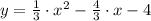 . Lastly, we must check if
. Lastly, we must check if
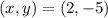 belongs to the function. If we know that
belongs to the function. If we know that
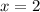 , then the value of
, then the value of
 is:
is:
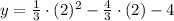
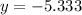
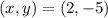 does not belong to the function, the real point is
does not belong to the function, the real point is
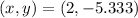 .
.