Is X 10 11 12 Y 18 18.6 19.2 proportional or non proportional

Question

asked Mar 13, 2022 15.2k views

1 Answer

Saolof · Answer 1 · 2022-03-17T14:15:39+0000

Given:

The table of values.

To find:

Whether the given table is a proportional or non proportional relation.

Solution:

If y is proportional to x, then

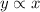
$y\propto x$

y=kx

(y)/(x)=k

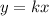
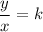
Where, k is the constant of proportionality.

Find the value of k for each ordered pairs in table. If the value of k is constant, then the relation is proportional.

The ordered pairs from the table are (10,18), (11, 18.6), (12,19.2). The values of k for each ordered pair is:

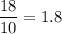
(18)/(10)=1.8

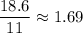
$(18.6)/(11)\approx 1.69$

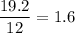
(19.2)/(12)=1.6

Since the value of k is not constant, therefore the given table represents a non proportional relationship.