Question

The walls of a bathroom 2,5 m long, 2,05 m

wide and 3 m high are to be covered with
tiles, each 15 cm by 15 cm. If a saving of
108 tiles is made on doors and windows,
how many tiles will be needed altogether?
Note that some tiles will have to be cut.)

Answer 1

The number of tiles needed is 1.105.
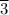`\overline 3` tiles

Explanation:

The given data on the dimensions of the bathroom wall are;

The length, l = 2.5 m

The width, w = 2.05 m

The height, h = 3 m

The dimension of the tiles with which the walls are to be covered = 15 cm by 15 cm

The dimensions of the tiles in meters = 0.15 m by 0.15 m

The number of tiles savings made on the doors and windows of the bathroom = 108 tiles

Let 'A' represent the surface area of the bathroom wall, we have;

A = h·w + h·w + l·h + l·h = 2·h·w + 2·l·h = 2·h·(w + l)

∴ A = 2 × 3 m (2.5 m + 2.05 m) = 27.3 m²

The surface area per tile, Tₐ = 0.15 × 0.15 = 0.0225

∴ Tₐ = 0.0225 m²/tile

The number of tiles needed, n = A/Tₐ - 108 tiles

∴ n = 27.3 m²/(0.0225 m²/tile - 108 tiles =
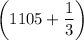`\left (1105+(1)/(3) \right )` tiles = 1.105.
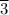`\overline 3` tiles

The number of tiles needed, n = 1.105.
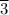`\overline 3` tiles.