Question

Need help with math problem if do get 5 star

Answer 1

Option A. 56 square units

Explanation:

Using the formula:

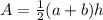`A = (1)/(2)(a+b) h` or
`A = (a+b)/(2) h`

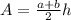
We substitute x with a and y with b:

x should be 3 units at the top, it has probably been cut off.

To work out b which should be the length at the bottom:

5+x+5 = 5+3+5 = 13

So b = 13

Now we substitute all values into the equation:

A = ((3+13)÷2)×7

A = (16÷2)×7

A = 8×7

A = 56

Therefore our answer is 56 square units

Answer 2

a) 56 square units

Explanation:

x = 3 (width - w)

y = 5 (base - b)

h = 7 (length and height - l and h)

• Area of a rectangle: l*w
• Area of a triangle: 1/2*b*h

For the triangles:

Area of a triangle: 1/2*b*h

A = 1/2 * 5 * 7

A = 1/2 * 35

A = 17.5

*Since there are two triangles, it would be 17.5 * 2 = 35

For the rectangle:

Area of a rectangle: l*w

A = 7 * 3

A = 21

Now, we add them together:

A = 35 + 21

A = 56 square units

Therefore, the area of the parallelogram is 56 square units

Hope this helps!