Answer:
Option D
Explanation:
Let the equation of the line given in the graph is,
y = mx + b
Here, m = Slope of the line
b = y-intercept
From the graph attached,
y-intercept = 4
Since, slope of the line passing through two points
 and
and
 is given by,
is given by,
m =
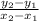
Therefore, slope of the line passing through (-2, 8) and (5, -6) will be,
m =
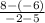
m =
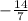
m = -2
Therefore, equation of the line will be,
y = -2x + 4
Equations given in the options A, B, and C are not matching with our equation.
Simplify the equation given in Option D,
y = -2(x - 5) -6
y = -2x + 10 - 6
y = -2x + 4
Therefore, Option D is the answer.