Question

In a Young's double-slit experiment the separation distance y between the second-order bright fringe and the central bright fringe on a flat screen is 0.0176 m, when the light has a wavelength of 425 nm. Assume that the angles are small enough so that is approximately equal to . Find the separation y when the light has a wavelength of 614 nm.

Answer 1

Y = 0.0254 m = 25.4 mm

Step-by-step explanation:

The formula for the fringe spacing in Young's Double-slit experiment is given by the following formula:

`Y = (\lambda L)/(d)`

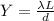
where,

Y = fringe spacing = 0.0176 m

λ = wavelength = 425 nm = 4.25 x 10⁻⁷ m

L = Distance between screen and slits

d = slit separation

Therefore,

`(0.0176\ m)/(4.25\ x\ 10^(-7)\ m) = (L)/(d)\\\\(L)/(d) = 41411.76`

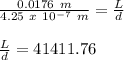
Now, for:

λ = 614 nm = 6.14 x 10⁻⁷ m

`Y = (6.14\ x\ 10^(-7)\ m)(41411.76)\\`

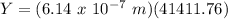
Y = 0.0254 m = 25.4 mm