Question

What does f mean in f(x)=3/2x+2?

Also do the two points 0,2 and 2,5 work with the equation?

Answer 1

f(x) means y

Points 0,2 and 2,5 work with the equation

Explanation:

f(x) means y

For 0, 2

y = 3/2(0) + 2

y = 2

For 2,5

y =3/2(2) + 2

y = 5

Answer 2

(0, 2) and (2, 5) are solutions to the function.

Explanation:

Given the following linear function:

`\displaystyle\mathsf{f(x)\:=\:(3)/(2)x\:+\:2 }`

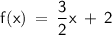
"f(x)" is a function notation.

"f" represents the function name. In function notations, you can use any letter to represent a function name. However, "f" is commonly used to express function notations.

"x" is your input variable. This is the x-values that you substitute into the function to get an output, or y-value.

f(x) is your output value.

We are also given the following points: (0, 2) and (2, 5).

In order to determine whether these points work with the given function, simply substitute their values into the function.

(0, 2) where x = 0, y = 2:

`\displaystyle\mathsf{f(x)\:=\:(3)/(2)x\:+\:2 }`

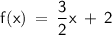
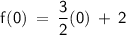`\displaystyle\mathsf{f(0)\:=\:(3)/(2)(0)\:+\:2 }` ⇒ The function notation, "f(0)" simply means the function of zero, which corresponds to the input value of zero (0).

Next, substitute the value of y = 2 into the function as an output value:

`\displaystyle\mathsf{2\:=\:(3)/(2)(0)\:+\:2 }`

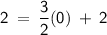
2 = 0 + 2

2 = 2 (True statement). This means that the point, (0, 2), is a solution to the given function. The point (0, 2) represent the given function's y-intercept, which is the point on the graph where it crosses the y-axis. The y-coordinate of 2 is the value of b in the slope-intercept form, y = mx + b.

(2, 5) where x = 2, y = 5:

We will do the same process for (2, 5):

`\displaystyle\mathsf{f(x)\:=\:(3)/(2)x\:+\:2 }`

`\displaystyle\mathsf{f(2)\:=\:(3)/(2)(2)\:+\:2 }`

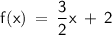
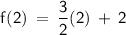
Substitute the value of y = 5 into the function as an output value:

`\displaystyle\mathsf{5\:=\:(3)/(2)(2)\:+\:2 }`

5 = 3 + 2

5 = 5 (True statement). This means that the point, (2, 5), is also a solution to the given function.