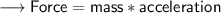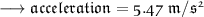Question

A car of mass 1200kg starting from 15m/s achieved the velocity of 66m/s ,If 6572N force is applied then find out time taken and acceleration.

Note:-

If p/q form arises find answer in decimals .


Read the question first then answer.


Spams/wrong/plagarised/short answers will be deleted on the spot .

​

Answer 1

`\large\boxed{\sf Time =9.30\ s }`

`\large\boxed{\sf Accl^n =5.47\ m/s^2 }`

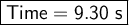
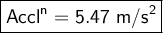
Step-by-step explanation:

Here it is given that a car of mass 1200kg has an initial velocity of 15m/s achieves a velocity of 66m/s . A force of 6572N was applied in order to increase the velocity and we need to find the time taken in doing so .

From Newton's Second Law of Motion ,

• The rate of change of momentum is directly proportional to the applied force in the direction of the force . Mathematically ,

`\sf\qquad\longrightarrow Force = \triangle p\\\\`

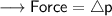
As momentum (p) = mass × velocity ,

`\\\sf\qquad\longrightarrow Force = (m(v-u))/(t) \\\\`

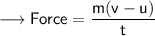
Here ,

• u = 15m/s
• v = 66m/s
• Force = 6572N

• On substituting the respective values ,

`\sf\qquad\longrightarrow 6572N = (1200kg ( 66- 15 )m/s )/(t)\\\\`

`\sf\qquad\longrightarrow 6572N = 1200kg * (51)/(t) \\\\`

`\sf\qquad\longrightarrow t = (1200×51)/(6572N ) \\\\`

`\sf\qquad\longrightarrow t =(61200)/(6572)s \\\\`

`\sf\qquad\longrightarrow \pink{\frak{ Time = 9.30\ s }}`

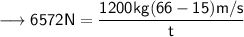
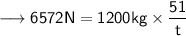
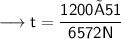
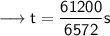
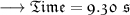
• For finding acceleration ,

`\sf\qquad\longrightarrow Force = mass * acceleration \\\\`

`\sf\qquad\longrightarrow accl^n =(Force)/(mass) \\\\`

`\sf\qquad\longrightarrow accl^n =(6572N )/(1200kg) \\\\`

`\sf\qquad\longrightarrow \pink{\frak{ acceleration= 5.47\ m/s^2}} \\\\`

`\rule{200}4`

Answer 2

acceleration: 5.48 m/s²

time taken: 9.3 seconds

Given:

• mass: 1200 kg
• force: 6572 N
• initial velocity: 15 m/s
• final velocity: 66 m/s

`\boxed{\sf acceleration = (Force)/(mass)}`
`\boxed{\sf time \ taken = (final \ velocity - initial \ velocity)/(acceleration) }`

using the formula's:

answer 1:

`\sf acceleration = (F)/(m)`

`\sf acceleration = (6572 )/(1200 )`

`\sf acceleration = 5.4766... \ m/s^2`

`\sf acceleration = 5.48 \ m/s^2` // rounded to nearest hundredth //

answer 2:

`\sf time \ taken = (\Delta v)/(a)`

`\sf time \ taken = (66 - 15)/(5.48)`

`\sf time \ taken = 9.3122 \ seconds`

`\sf time \ taken = 9.3 \ seconds` // rounded it to nearest tenth //