Question

In an article by researchers Meehl and Rosen (Psychological Bulletin, 1955), a diagnostic test was examined for detecting psychological adjustment in soldiers. A positive result indicates the solder is mal-adjusted whereas a negative result indicates well-adjusted. For soldiers known to be well-adjusted, the test gives a positive result 19% of the time. For soldiers known to be mal-adjusted, the test gives a positive result 55% of the time. The researchers believed that 5% of all soldiers are mal-adjusted. Suppose a soldier is selected at random and they test positive. What is the probability that soldier is mal-adjusted

Answer 1

0.1322 = 13.22% probability that the soldier is mal-adjusted.

Explanation:

Conditional Probability

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

In which

P(B|A) is the probability of event B happening, given that A happened.

`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

In this question:

Event A: Positive test

Event B: Soldier is mal-adjusted.

Probability of a positive test:

55% of 5%(mal-adjusted).

19% of 100 - 5 = 95%(well adjusted). So

`P(A) = 0.55*0.05 + 0.19*0.95 = 0.208`

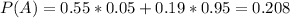
Probability of a positive test and soldier being mal-adjusted.

55% of 5%. So

`P(A \cap B) = 0.55*0.05 = 0.0275`

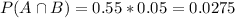
What is the probability that the soldier is mal-adjusted?

`P(B|A) = (P(A \cap B))/(P(A)) = (0.0275)/(0.208) = 0.1322`

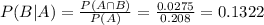
0.1322 = 13.22% probability that the soldier is mal-adjusted.