Answer:
A sample size of 1380 should be obtained.
Explanation:
Minimum sample size:
The minimum sample size is of:
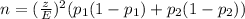
In which z is the critical value, related to the confidence level, E is the desired margin of error,
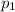 and
and
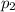 are the proportions.
are the proportions.
99% confidence level
So
 , z is the value of Z that has a pvalue of
, z is the value of Z that has a pvalue of
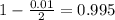 , so
, so
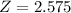 .
.
Estimates of 22.6% male and 18.1% female from a previous year
This means that
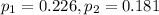 .
.
Within 3 percentage points, minimum sample size:
This is n for which
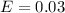 . So
. So
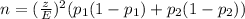
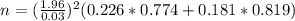
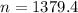
Rounding up:
A sample size of 1380 should be obtained.