Answer:
Part A
The worth of the stock in 7 years is approximately $145.68
Part B
The worth of the stock in 2016 is approximately $62.48
Part C
f(7) = 85 × (1 + 0.08)⁷
Part D
85 × (1 + 0.08)⁻⁴
Explanation:
Question
"The value of the πPhone πhas increased 8% each year for the past several years. In 2020, the stock was worth $85. What will the stock be worth in 7 years? What was the stock worth in 2016? Equation: 7 years from now? in 2016?"
The equation for the growth of the stock to a final amount f(x) is given by the general equation for exponential growth as follows;
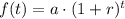
Where, in relation to the stocks;
f(t) = The value of the stocks after 't' years
a = The initial amount of the stocks
r = The rate of growth of the stock = 8%
t = The number of years of growth
Part A
The worth of the stock in 7 years, f(7), is found as follows;
The (initial) value of the stock in 2020, a = $85
The rate of increase in the value of the stock = The growth rate of the stock, r = 8% = 8/100 = 0.08
The number of years of growth, t = 7 years
We get;
f(7) = 85 × (1 + 0.08)⁷ ≈ 145.68
The worth of the stock in 7 years, f(7) ≈ $145.68
Part B
The value of the stock in 2016 is found as follows;
The number of years between 2020 and 2016 = 2016 - 2020 = -4 (years)
Here, let 'f(t)' represent the value of the stock in 2016, and therefore, we have;
t = -4 years
r = 8% = 0.08
a = The value of the stock in 2020 = $85
Plugging in the values gives;
f(-4) = 85·(1 + 0.08)⁻⁴ = 85 × (1.08)⁻⁴ ≈ 62.48
The value of the stock in 2016, f(-4) ≈ $62.48.
Part C
The equation for 7 years from now is f(7) = 85 × (1 + 0.08)⁷
Part D
The equation for 2016 is f(-4) = 85 × (1 + 0.08)⁻⁴.