Question

What is the volume of this triangular prism?

Answer 1

`\displaystyle V _{ \text{prism}} = 270 {cm}^(3)`

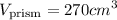
Explanation:

we are given a triangular prism

we want to figure out the Volume of the given Prism

remember that,

`\rm\displaystyle V _{ \text{prism}} = [\text{base area}] * l`

since the base is triangle the formula of base area should be the formula of the area of a triangle

thus,

`\rm\displaystyle V _{ \text{prism}} = [(1)/(2)* b* h] * l`

substitute

`\rm\displaystyle V _{ \text{prism}} = [(1)/(2)* 9 * 15]* 4`

remove parentheses:

`\displaystyle V _{ \text{prism}} = (1)/(2)* 9* 15* 4`

reduce fraction:

`\displaystyle V _{ \text{prism}} = 9* 15* 2`

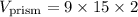
simplify multiplication:

`\displaystyle V _{ \text{prism}} = 270`

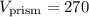
hence, the Volume of the given prism is 270cm³

Answer 2

Given:

perpendicular [p]=9cm

base[b]=15cm

length[l]=4cm

we have

the volume of this triangular prism=area of traingle. ×length=1/2 p×b×l

=½×9×15×4=270cm³

270cm³ is a required volume.