Answer:
The rocket will hit the ground after about 11.63 seconds.
Explanation:
A rocket is launched from a tower. Its height y in feet after x seconds is modeled by the equation:

We want to determine the time at which the rocket will hit the ground.
If it hits the ground, its height y above the ground will be 0. So, we can set y = 0 and solve for x:
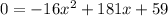
Solve the quadratic. Factoring is too tedious or impossible, and completing the square is also very tedious. So, we can use the quadratic formula:

In this case, a = -16, b = 181, and c = 59. Substitute:
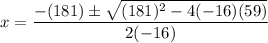
Evaluate:
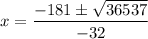
Simplify:
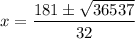
Hence, our solutions are:

Since time cannot be negative, we can ignore the second solution.
So, the rocket will hit the ground after about 11.63 seconds.