Answer:
(1,-1)
Explanation:
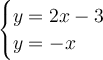
Since both are y-isolated equation. We can combine both.
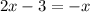
Since we want to solve for x-term, we need to isolate x-term.
So we move from -x which is the right side to x.
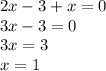
But we are not done yet. Solving system of equations, you must solve for y-term as well. (Because we have to answer in ordered pairs which are (x, y))
Substitute x = 1 in any given equations but I will substitute x = 1 in y = -x
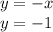
Since we have got y-value. Therefore the answer is (1,-1)