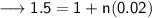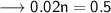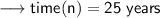Answer:
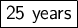
Explanation:
Here it is given that the initial population of a town is 2,000 . And it increases at rate of 2% per year . We need to find out in what time the population will become 3,000 . As we know that ,
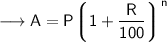
where ,
- A is the final population .
- P is the initial population .
- R is the rate of growth .
- n is the number of years .

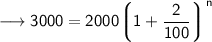
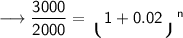
Now from Binomial Theorem , we know that ,

- if x << 1 . Hence here 0.02 <<1 .