Question

Find AB. Round to the nearest tenth if necessary.
​

Answer 1

I think the right answer would be 7

Explanation:

We got-ta use the equation: CA * BA = DA²

CA = 15 + BA

DA² = 8² = 64

=> (15 + BA) BA = 64

Subtract 15 from each side:

BA(BA) = 49

BA² = 49

Now take the square root of each side

BA = 7

Hope this helps!

Answer 2

The length of AB is approximately 19.13 units (rounded to the nearest tenth).

To find the length of AB, we can use the Power of a Point Theorem. This theorem states that for any point P outside a circle, the product of the lengths of the two segments that a secant line (or a chord extended) divides the circle into is equal. Mathematically, if
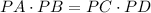`\(PA \cdot PB = PC \cdot PD\)`, then point P lies on the circle.

In this case, let point P be the extension of line AB to line AC. The segments are PA = AB and PB = BC. The other segments are PC = PD = AC.

So, we have:

`\[AB \cdot BC = AC \cdot AC\]`

Substitute the given values:

`\[AB \cdot 15 = AC \cdot AC\]`

Given that AD = 8, we can find AC using the Pythagorean Theorem:

`\[AC = √(AD^2 + CD^2) = √(8^2 + 15^2) = √(64 + 225) = √(289) = 17\]`

Now, substitute this value back into the equation:

`\[AB \cdot 15 = 17 \cdot 17\]`

`\[AB = (17 \cdot 17)/(15) \approx 19.13\]`

Therefore, the length of AB is approximately 19.13 units (rounded to the nearest tenth).