Question

Some fireworks are fired vertically into the air from the ground at an initial speed of 80 feet per second. The equation for this object's height h(t) at time t seconds after launch is h(t) = -16t2 + 80t. a. Find the vertex. Explain what this means in the context of the situation

Answer 1

Vertex (-2.5,-300)

Explanation:

We are given with h(t)=-16 t^2 +80 t. We are asked to find the vertex.

X-coordinate of vertex =
`(-b)/(2a)`

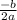
Where 'a' is coefficient of
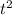`t^(2)` term

'b' is coefficient of 't' term.

From given function, a= -16

b=80

So,

x-coordinate of vertex =
`(-80)/(2(-16))`

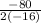
=-2.5

y-coordinate of vertex =
`-16(-2.5)^(2) +80(-2.5)`

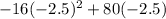
Simplify it,

=-100 -200

= -300

So, vertex is at (-2.5, -300).