Question

The volume of a cylinder is 542pi in?. The height of the cylinder is 16 in. What is the radius of the cylinder to the nearest hundredth?

The volume of a sphere is 5,055pi m. What is the surface area of the sphere to the nearest square meter?

Answer 1

I. Radius, r = 5.82 in

II. S.A of a sphere = 19.61 meters

Explanation:

Given the following data;

I. Volume of cylinder = 542π in³

Height = 16 in

To find the radius of the cylinder;

Volume of cylinder = πr²h

542π = πr²*16

Dividing both sides by π, we have;

542 = 16r²

r² = 542/16

r² = 33.875

Radius, r = 5.82 in

II. Volume of sphere = 5.055π m³

To find the surface area of the sphere;

First of all, we would find the radius of the sphere.

`Volume \; of \; a \; sphere = \frac {4}{3} \pi r^(3)`

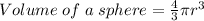
Substituting into the formula, we have;

`5.055 \pi = \frac {4}{3} \pi r^(3)`

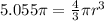
Cross-multiplying, we have;

`5.055 \pi * 3 = 4 \pi r^(3)`

`15.165 \pi = 4 \pi r^(3)`

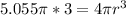
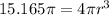
Dividing both sides by 4π, we have;

`3.79 = r^(3)`

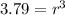
Taking the cube root of both sides, we have;

r = 1.56 m

Next, we find the surface area (S.A) of the sphere;

S.A of a sphere = 4πr

S.A of a sphere = 4*3.142*1.56

S.A of a sphere = 19.61 meters