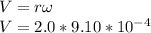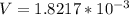Question

A woman of mass 55 kg stands on the rim of a frictionless merry-go-round of radius 2.0m and rotational inertia 1250 kg-m2 that is not moving. She throws a rock of mass 350g horizontally in a direction that is tangent to the outer edge of the merry-go-round. The speed of the rock, relative to the ground, is 2.0m/s. Afterward, what are (a) the angular speed of the merry-go-round and (b) the linear speed of the woman

Answer 1

a)
`\omega=9.10*10^(-4)rad/sec`

b)
`V=1.8217*10^(-3)`

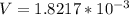
Step-by-step explanation:

From the question we are told that:

Mass of woman
`M_w=55kg`

Radius of merry go round
`r=2.0m`

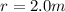
Rotational inertia
`i= 1250 kg-m2`

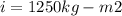
Mass of rock
`M_r=350g \approx 0.350kg`

Speed of rock
`V_r=2.0m/s`

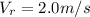
Tangent angle to the outer edge
`\theta=1`

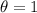
a)

Generally the equation for conservation of momentum is mathematically given by

`M_r(ucos\theta)r=(I+M_wr^2)\omega`

`0.350(2.0cos1)(2.0)=(1250+(55)(2.0)^2)\omega`

`1.3998=1470\omega\\\omega=(1.339)/(1470)`

`\omega=9.10*10^(-4)rad/sec`

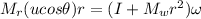
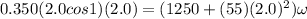
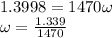
b)

Generally the equation for linear speed V is mathematically given by

`V=r\omega\\V=2.0*9.10*10^(-4)`

`V=1.8217*10^(-3)`