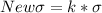Question

Consider the following. 12, 7, 18, 23, 24, 27 Compute the population standard deviation of the numbers. (Round your answer to two decimal place.) (a) Double each of your original numbers and compute the standard deviation of this new population. (Round your answer to two decimal place.) (b) Use the results of part (a) and inductive reasoning to state what happens to the standard deviation of a population when each data item is multiplied by a positive constant k.

Answer 1

(a)
`\sigma = 7.04`

(b)
`\sigma = 14.1`

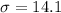
(c) The population standard deviation is multiplied by k

Explanation:

Given

`Dataset: 12, 7, 18, 23, 24, 27`

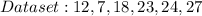
Solving (a): The population standard deviation

Start by calculating the mean

`\mu = (\sum x)/(n)`

`\mu = (12+7+18+23+24+27)/(6)`

`\mu = (111)/(6)`

`\mu = 18.5`

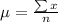
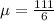
The population standard deviation is:

`\sigma = \sqrt{(\sum(x - \mu)^2)/(n)}`

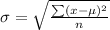
This gives:

`\sigma = \sqrt{((12-18.5)^2 + (7 - 18.5)^2 + (18-18.5)^2 + (23-18.5)^2 + (24 - 18.5)^2 + (27 - 18.5)^2)/(6)}`

`\sigma = \sqrt{(297.5)/(6)}`

`\sigma = √(49.5833)`

`\sigma = 7.04`

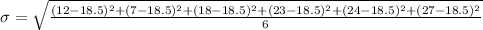
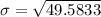
Solving (b): Double the dataset and calculate the new population standard deviation

The new dataset is:

`Dataset: 24, 14, 36, 46, 48, 54`

Start by calculating the mean

`\mu = (\sum x)/(n)`

`\mu = (24+ 14+ 36+ 46+ 48+ 54)/(6)`

`\mu = (222)/(6)`

`\mu = 37`

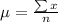
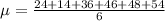
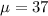
The population standard deviation is:

`\sigma = \sqrt{(\sum(x - \mu)^2)/(n)}`

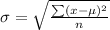
This gives:

`\sigma = \sqrt{((24-37)^2 +(14-37)^2 +(36-37)^2 +(46-37)^2 +(48-37)^2 +(54-37)^2)/(6)}`

`\sigma = \sqrt{(1190)/(6)}`

`\sigma = √(198.33)`

`\sigma = 14.1`

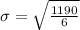
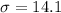
Solving (c): What happens when the dataset is multiplied by k

In (a), we have:

`\sigma = 7.04`

In (b), when the dataset is doubled,

`\sigma = 14.1`

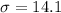
This implies that when the dataset is multiplied by k, the population standard deviation will be multiplied by the same factor:

i.e.

`New \sigma = k * \sigma`