Question

A cyclist intends to cycle up an 8 degrees hill whose vertical height is 150 m, with constant speed. If each complete revolution of the pedals moves the bike 6 m along its path, calculate the average force that must be exerted on the pedals tangent to their circular path. Neglect work done by friction and other losses. The pedals turn in a circle of diameter D = 30 cm. The total mass of the cyclist and his bike is 150 kg.

Answer 1

Answer:
`1302.25\ N`

Step-by-step explanation:

Given

Inclination of hill
`\theta=8^(\circ)`

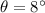
Each revolution moves 6 m along its path

Diameter of the circle
`D=30\ cm`

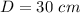
the total mass of the cyclist and his bike
`M=150\ kg`

Suppose a single pedal cyclist gain a vertical height of
`\Delta y`

`\therefore \sin \theta =(\Delta y)/(6)\\\\\Delta y=6\sin 8^(\circ)`

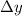
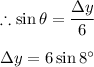
Average force cause to gain in Potential energy

`\therefore\ F_(avg)\cdot 2\pi r=Mg\Delta y\\\Rightarrow F_(avg)=(150* 9.8* 6\sin 8^(\circ))/(2\pi\cdot 0.15)\\\\\Rightarrow F_(avg)=1302.25\ N`

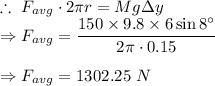
Thus, the average force is
`1302.25\ N`